

RESEARCH LINES:
ELECTRONIC AND ELASTIC PROPERTIES OF GRAPHENE
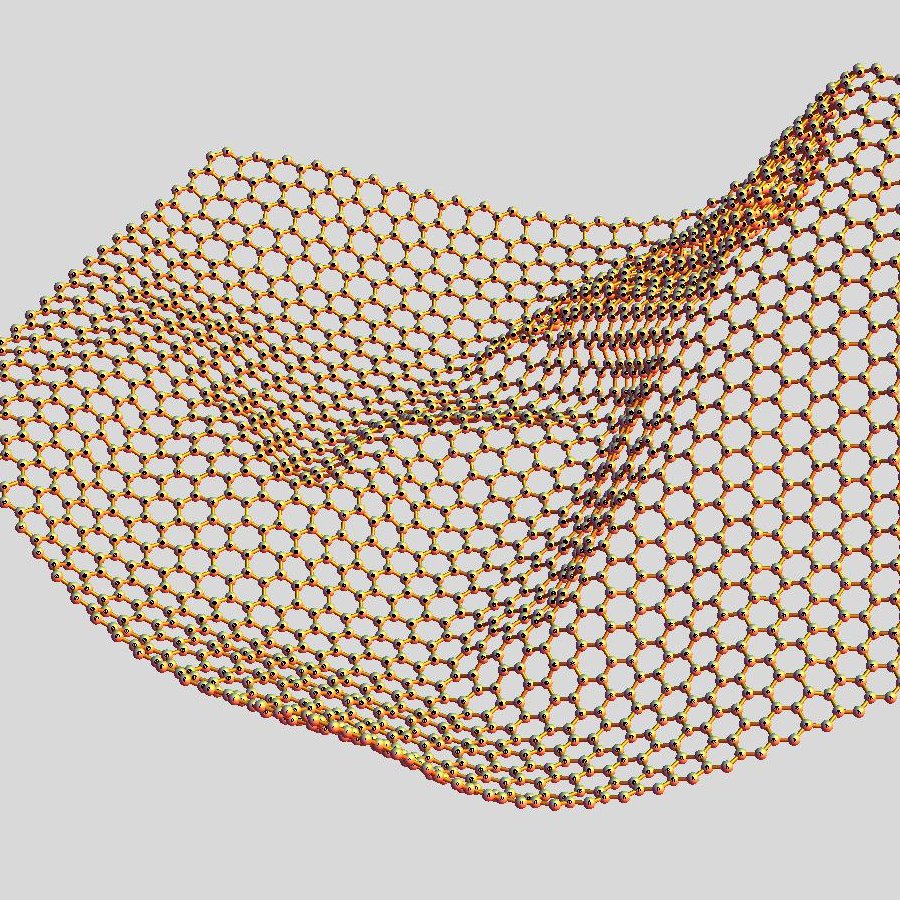
In recent years we have continued our investigation of the properties of graphene. This material, made of a single layer of carbon atoms with honeycomb structure, is currently attracting much attention in the condensed matter community, both for the theoretical challenges it presents as well as for its potential for technological application. We have pursued different lines of research, devoted in particular to understand the role of the electronic correlations and to explain the intrinsic corrugation of graphene from the interplay of electronic and elastic degrees of freedom.
Regarding the study of the electronic correlations, we have investigated the development of a gapped phase in the low-energy theory of Dirac quasiparticles with long-range Coulomb interaction. The case of Quantum Electrodynamics in 2+1 dimensions can serve as a good example of this phenomenon, by which the original U(N) chiral symmetry of the theory with N massless two-component Dirac fermions is spontaneously broken below a critical number of flavors. We have shown that, in the large-N approximation, an excitonic gap can only appear in low-energy theory of graphene below N = 32/π2, that is exactly half the value found in Quantum Electrodynamics at the same number of dimensions. This implies that, given the physical value N = 4, the electronic spectrum should remain gapless even for the largest interaction strength attained in suspended graphene. This is in agreement with the fact that no evidence of transition to an insulating state has been found in experiments carried out with free-standing samples. Anyhow, we have also given evidence that the chiral symmetry should be broken at sufficiently small values of N, as the order parameter of the transition turns out to diverge at a certain critical coupling in the ladder approximation. A natural prediction from our analysis is that the gapped phase should emerge at some point in the way from N = 4 to N = 1. The spin degree of freedom can be frozen for instance by applying a magnetic field, and it is actually very appealing to think that the metal-insulator transition observed in that case in graphene may rest on this effect of chiral symmetry breaking. It remains to be seen whether quenching the remaining Dirac-valley degree of freedom could also lead to an insulating state for accessible values of the interaction strength, in accordance with the results of our study.
On the other hand, graphene can be considered as a prototype of electronic crystalline membrane, where the mobile electrons are strongly coupled to the phonons of the two-dimensional lattice. A remarkable and unexpected property observed in graphene is its tendency to develop ripples, or long wavelength modulations of the out-of-plane displacements, by which the system freezes into a corrugated average configuration. Ripples are an important feature of graphene, as they are expected to have a significant impact on transport properties, and their origin has been heavily debated. In exfoliated graphene, ripples are correlated to some extent with the irregularities of the substrate. But it has been also observed, mainly from experiments in suspended graphene, that they also arise in part as an effect intrinsic to the crystalline membrane.
In our work, we have focused on the behavior of graphene as an electronic membrane in order to investigate the rippling instability. A key observation is that the studies of more conventional membranes have shown that these have in general a flat phase with increasing rigidity over large length scales. We have then argued that the novel factor peculiar of graphene, the mobile electrons, must play an important role in the ripple formation mechanism. Within the framework of quantum many-body theory, we have devised a self-consistent approach supplemented by a renormalization group method to evaluate the contribution of the electrons to the phonon self-energy corrections. The nonperturbative character of this approach has allowed us to find a critical value of the electron-phonon coupling at which the effective bending rigidity of the membrane vanishes. This effect takes place at zero temperature and corresponds then to the transition to a new ground state of the system, characterized by the spontaneous breakdown of the translational symmetry of the flat membrane. We have shown that this follows a mechanism similar to that responsible for the condensation of the Higgs boson field in elementary particle physics, with an order parameter given in graphene by the square of the gradient of the flexural phonon field. The effective potentials for both quantum fields (the vertical displacement in graphene, the Higgs field in relativistic physics) have actually the same typical "mexican-hat" shape, which makes the unstable state at the top to spontaneously decay by rolling down to a lowest-energy state with broken symmetry (the aggregate of ripples in one case, the Higgs condensate filling the vacuum in the other). We have shown that the analogue goes even further, since graphene has a control parameter (the tension of the membrane) that plays the same role as the mass square for the Higgs field, driving the instability in the case of compression (negative tension). Choosing suitable values of the parameters, we have seen that the proposed model leads to a predicted buckled phase which is consistent with experimental observations of the ripples, illustrating another way to employ graphene as a test ground of fundamental concepts in theoretical physics.
The quantum many-body problem is rooted in the difficulties of finding exact solutions, either analytically or numerically, to the Schrödinger equation for many interacting particles. This limitation has stimulated the development of approximations such as Hartree-Fock, Bardeen-Cooper-Schrieffer (BCS), Hartree-Fock-Bogoliubov or Bogoliubov-de Gennes, density functional theory, etc. These methods and their extensions to include correlations and fluctuations beyond mean field were fundamental tools for the understanding of a great variety of physical phenomena in microscopic and mesoscopic systems, ranging from atomic nuclei, atoms and molecules to artificial quantum systems such as quantum dots, nanograins, and ultracold gases. However, the validity of these approximate methods is questionable for low dimensional strongly correlated systems or critical systems close to a phase transition, where new phenomena occur (high-temperature superconductivity, fractional quantum Hall effects, unconventional phases of ultracold atoms and nuclear matter , etc...) that can not be explained at the mean field level. Some of the grand theoretical challenges to face are: what are the minimal conditions for these strongly correlated phases to appear?, what are the corresponding phase transitions and order parameters and how can they be quantified? We, in general address this question through a two-pronged strategy. On the one hand we generalize exactly solvable and fully integrable quantum models and we develop new techniques for large scale numerical calculations. These two lines of research are aimed at the same goal: obtaining new insights on strongly correlated quantum systems, either through exact numerical solutions or through approximate solutions with high and controlled accuracy. On the other hand, we will study dynamical phenomena associated with quantum transport and quantum decoherence for critical systems or for systems coupled to a critical bath. The objectives of our research can be classified into three great themes: a) Exactly solvable models, b) Large scale numerical calculations for strongly correlated systems, and c) Quantum transport and quantum decoherence.
Correlations in quantum many-body systems are hard to grasp due to the counterintuitive nature of quantum physics. Exactly solvable models (ESM) have provided important insights that could not be obtained from semi-classical or mean-field approximations. A particular example of an exact solution that has had an enormous impact in the field is the ansatz used by Bethe in 1931 to find the exact solution of the Heisenberg spin Hamiltonian. In 1963, six years after the publication of the BCS theory that revolutionized the field of superconductivity and superfluidity, Richardson showed that the pairing Hamiltonian from BCS theory was exactly solvable. For decades this work remained almost unnoticed, until our group rediscovered the solution in 2000 in an effort to describe the superconductor to normal transition in ultrasmall aluminum grains. Over the past 8 years this has spurred a renewed interest in the Richardson's exact solution and the development of new ESM based on the Richardson ansatz. Combining the Richardson ESM and the integrable Gaudin spin model (Gaudin magnet), we have derived new families of Richardson-Gaudin (RG) ESM. The RG models describe pairing correlations in fermionic systems (based on a SU(2) algebra) or bosonic systems (SU(1,1) algebra). They are relevant for superconducting grains, nuclear structure, electrons in 2D lattices, trapped ultracold gases, etc... More recently we have succeeded in extending the RG models to algebras of higher rank, in particular the isovector pairing represented by the rank-2 SO(5) algebra (Phys. Rev. Lett. 96, 072503 (2006)) and the isovector-isocalar proton-neutron pairing represented by the rank-4 SO(8) model (Phys. Rev. Lett. 99, 032501 (2007)).
Lately, using the exact solution of the SO(8) model of proton-neutron pairing, we have studied the precision of the BCS and number projected BCS approximations in finite size systems. By taking into account finite size effects and solving the Richardson-Gaudin equations for large systems we showed that the proton-neutron BCS approximation is exact in the thermodynamic limit. We studied the p-wave pairing realization of the hyperbolic Richardson-Gaudin model describing spinless cold atom systems. These systems are characterized by a topological phase, and differently from s-wave systems that have a quantum phase transition between a superconducting phase and a Bose-Einstein condensate of dipolar molecules. The exact solution allowed us to establish that the phase transition is of 3º order and to interpret it as a confinement-deconfinement transition as viewed from the exact wave function.
b) LARGE SCALE NUMERICAL CALCULATIONS FOR STRONGLY CORRELATED SYSTEMS
Low dimensional quantum systems dramatically expose the effects of interactions on quantum correlations, as exemplified by the phenomenom of spin-charge separation in one-dimensional fermionic lattices. The size of the Hilbert space of a many-body lattice system increases exponentially with the number of lattice sites. Hence any exact diagonalization is restricted to very small systems. The Density Matrix Renormalization Group (DMRG) is an iterative approach that can be used to calculate the energy and correlation functions for the ground state and a few excited states of a one-dimensional lattice model. The algorithm is designed to find an optimal truncation of the Hilbert space and can produce virtually exact results for very large one-dimensional lattices (depending on the model up to 400 sites or more). We apply our DMRG code to ultracold gases composed of bosonic and/or fermionic atoms confined in artificial optical lattices. These systems have become an important instrument for investigating the physics of strong correlations as their parameters and dimensionality can be tuned with very high precision and control. The different hyperfine states of the atom can be used to study the phases of high-spin fermions, for example. On a different respect, the DMRG or matrix-product-state methods have shown to be much less succesfull for strongly correlated systems in 2D lattices. This is mainly due to the need for defining a 1D path in the 2D lattice, which induces long-range interactions and fermion exchange effects. Dynamical mean-field methods (DMFT) are considered to be the state-of-the-art for 2D systems, but they have limitations because they basically map the whole system onto a single cluster and therefore cannot be applied to non-uniform systems. We believe that these issues can be overcome with a new cluster method that we recently proposed: the hierarchical mean-field theory (HMFT). It treats the intracluster dynamics exactly such that short-range correlations are accurately described. By mapping odd-number cluster states onto composite fermions one preserves the long-range fermionic exchange correlations, even when these composite fermions are treated at the mean-field level. By extending the cluster size one obtains a systematic route to the exact solution. Compared to the (DMFT), the HMFT has the advantage that it is variational in the energy and can be applied to non-uniform systems such as trapped fermionic gases. In 3D, correlations are not so prominent and it is expected that educated mean-field approaches like Hartree-Fock-Bogoliubov could describe in a good qualitative manner the physics of mesoscopic systems. Over the last decades, this line of research has been pursued in nuclear structure calculations developing extremely efficient codes that can account for symmmetry breaking phases like superfluidity and shape deformations. These codes are devoted to explore nuclear regions far from stability with large proton-neutron asymetries and of relevance for astrophysical processes. Quantum Monte Carlo methods (QMC) offer an alternative approach that we are developing. They are based on a sampling of many-particle trajectories in imaginary time. The statistics of these trajectories can be related to the thermodynamical partition function and quantities that can be derived from that. At large imaginary times (i.e. zero temperature) the trajectories converge to the exact ground state of the quantum system. While very successful for bosonic systems and one-dimensional fermionic systems, the application of QMC to fermionic systems in 2D and 3D has remained cumbersome due to trajectories with negative weights. A way to avoid this sign problem is to restrict the trajectories to regions of phase space that have a positive overlap with a given schematic wave function. This fixes the nodes of the wave function that one tries to solve, and the accuracy of the QMC result will depend on how well the nodes of the schematic wave function approximate the nodes of the exact ground state.
By means of the Hierarquical Mean Field approximation developed in our group, we have studied the J-Q model of quantum magnetism, recently proposed as an example of system having a deconfined quantum critical point. In our representation we find a 2º order quantum phase transition between a Neel state and scalar plaquette phase, contradicting previous numerical results and starting a debate about the characteristics of this quantum phase transition. Moreover, there are discrepancies about the magnitude of the critical coupling that differs in one order of magnitude between the different treatments.
We have also studied the separation between the charge and color degrees of freedom in systems of ultracold trapped fermionic atoms with three different hyperfine states generalizing for these systems the phenomenon of spin-charge separation that appears in strongly correlated one-dimensional systems. We have performed large-scale simulations by means of the time-dependent density matrix renormalization group algorithm. We have observed the different velocities of displacement of the charge and color excitations. We have explored the differences between attractive and repulsive interactions and we have studied the effects of the anisotropy of the interactions.
c) QUANTUM TRANSPORT AND QUANTUM DECOHERENCE
Technological advances have allowed the experimental realization of nanostructures smaller than the phase coherence length where the applicable theory is quantum mechanics (mesoscopic systems). Due to the continuous effort in the miniaturization of electronic devices a perfect understanding of quantum transport is needed to keep up advances in nanotechnology, allowing the design of smaller, more efficient, and less energy consuming electronic devices. Moreover, the appearance of new physical phenomena will spur the development of new applications. In these low-dimensional nanostructures, a many-body description is unavoidable. Theoretical research in strongly correlated models is fundamental for understanding the effects of the interaction. In practice, the new devices must work in non-equilibrium conditions, and we need to understand their dynamical and transport properties. In the case of non-interacting problems, the Landauer-Büttiker formulation allows the calculation of the current flowing through a mesoscopic system, which is proportional to the transmission coefficient. In the presence of the interaction, although some theoretical advances have been made, there is no known practical and universally valid solution. In this context we have developed the embedding method that has been very successful for the calculation of the conductance of one-dimensional strongly correlated systems. We have been able to justify the validity of a Landauer-like approximation with an interaction-dependent transmission under certain conditions. These results will allow us to study the transport properties of molecules, quantum wires, and nanotubes. Another important feature to face is the phenomenon of decoherence. Due to the interaction with the environment, the system loses (at least) part of its quantum coherence and correlations. The consequence is that the system transites to a macroscopic state, in which the quantum phenomena become negligible and all its mesoscopic features are lost. Therefore, the study of quantum decoherence is a very active research field. Our group has been recently involved in the study of the influence of the environment's dynamical regime and the presence of quantum phase transitions in the decoherence induced in a small central system composed by one or two qubits. Our main aim in this subject is to analyze the effect of the order of the phase transition and the multipolarity of the bosons composing the environment in the decoherence induced in the small central system. For this purpose we rely both on large scale numerical calculations and analytical methods, time-dependent mean-field and exact solutions on the integable limits. Another aspect that will encompass transport and decoherence is the use of the recently developed Time-dependent Density Matrix Renormalization Group (TDDMRG) as numerical method to treat non-equilibrium properties of strongly-correlated systems.
Recently, we have calculated the full conductance distribution of disordered quantum wires in the presence of a time-periodic field. Our calculations were based upon Floquet theory and the scaling theory of localization. The effects of the field in the conductance statistics are very strong, specially in the high-frequency limit were the conductance distribution shows a sharp cut. In this regime, the conductance can be written as the product of two terms, one frequency-dependent and one field-independent with the information of the disorder of the system. We used the solution of the Mel'nikov equation to calculate the distribution of the conductance for an arbitrary strength of teh disorder. For low frequencies, we find that the conductance distribution and the Floquet mode correlations can be described by the solution of the Dorokhov-Mello-Pereyra-Kumar equation with an effective number of channels. In the regime of strong localization, that can be induced either by the disorder or by the driving the conductance distribution is a log-normal distribution. We have verified our theoretical results with numerical calculations in the Anderson model.
We have also studied non-equilibrium dynamics and thermalization in an interacting spin system, which transites from integrability to chaos. The integrable limit consists of the Elliptic Gaudin model; the transition towards chaos was achieved by means perturbing the former. The main results obtained are the following: a) quantum chaos does not guarantee thermalization; b) in spite of it is possible to find several constants of motion in the non-integrable cases, they store less information about the initial state than in the integrable case, and c) the mechanisms of thermalization are in agreement with the Eigenstate Thermalization Hypothesis.